Invited Speakers

Miriam Amit and Shlomi Dolev, Ben Gurion University, Beer Sheva, Israel

TBA

Gershon Elber, Computer Science Department, Technion, Haifa, Israel

Science Art Synergy

The art work of M. C. Escher needs no introduction. We have all learned to appreciate the impossibilities that this master of illusion's artwork presents to the layman's eye. Nevertheless, it may come as a surprise for some, but many of the so-called 'impossible' drawings of M. C. Escher can be realized as actual physical, tangible, three-dimensional objects, in many cases using layered manufacturing technologies. These tangible objects will resemble the Escher's drawings when viewed from a certain viewing direction.

In this talk, I will discuss several art-related applications but in the context of computer aided geometric design and manufacturing software tools, extrapolating on drawings and art-work made by Escher and many others. We will portray several unique geometric modeling tools that enable one to synthesize many of these so called ‘impossible’ and illusive objects, only to be fabricated by layered manufacturing technologies (3D printing), among others.

and http://www.cs.technion.ac.il/~gershon/BeyondEscherForReal

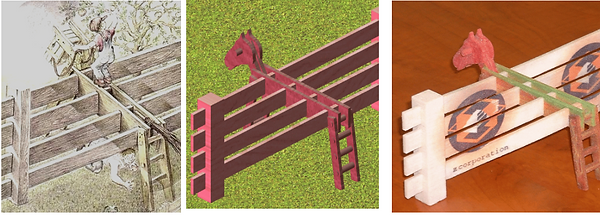
Left: The “Garden Fence” by Sandro Del-Prete. Middle: The computer Model.

Right: a manufactured model using a layered manufacturing technology.

Michal Tabach, Tel Aviv University, Israel

Teacher competencies as the key to integrating digital resources into the mathematics classroom

In the 21st century, mathematics teaching can no longer disregard either technological tools or students’ use of technology outside of school. Yet the vast majority of mathematics teachers studied mathematics as K-12 students in a paper-and-pencil environment, and their training to become teachers did not place major emphasis on digital resources. The apparent solution to this problem is to offer professional development courses that focus specifically on integrating digital resources into the mathematics classroom. Yet, research shows that such professional development efforts rarely lead to classroom implementation. One possible explanation is that these efforts are not directed at the teachers’ needs. What, then, do teachers need to be able to integrate technology in the classroom? Are there standards for such competencies? What theoretical tools are available to examine these competencies? What are the implications for teacher education? The talk will focus on these and similar issues.

Ilias Kotsireas, Wilfrid Laurier University, ON, Canada

Computational Discovery and Experimentation in Mathematics

In the past few decades, researchers have been using extensively various computer science technologies, such as artificial intelligence, data mining, high-performance computing, symbolic computation, computer graphics and so forth, as active tools in mathematical research. We will present several of these technologies and their associated tools and methodologies and will illustrate them with examples. This fertile interaction has allowed researchers to formulate conjectures suggested by experiments, put forward hypotheses supported by data and subsequently prove rigorous mathematical results, based on experimentation. We will also describe how this trend materializes in our own research in the area of combinatorial designs.

Zoltán Kovács, The Private University College of Education of the Diocese of Linz, Linz, Austria

Automated discovery in planar geometry

The ancient Greek mathematicians collected most of the knowledge we already have on planar Euclidean geometry. But the way we teach this topic has been changed dramatically over the last decades. First, using dynamic geometry in the classroom initiated discovery by experimenting: thousands of conjectures can be stated only by constructing and dragging. Second, computer algebra initiated obtaining proofs for these conjectures, even in the classroom. Third, mixing dynamic geometry and computer algebra opened the road to obtain conjectures and their proofs at the same time. Finally, automated discovery can even search for interesting theorems related to certain objects in a given construction.

In the talk some recently available systems will be presented that support the novel approaches, including GeoGebra Discovery, an experimental tool dedicated to automated reasoning in planar Euclidean geometry.

Attila Szabo, Stockholm University, Sweden

Does one have to be good in mathematics in order to be creative in the subject? Importantly, is it possible to use computer programming in order to develop mathematical talent and creativity?

The lecture presents some perspectives on mathematical talent and creativity in an educational context. Further, the lecture discusses ways of nurturing mathematical talent and creativity by using computer programming in school mathematics. Moreover, programming tasks, aimed to develop pupils’ problem-solving ability, are presented.

SHORT BIOS

Gershon Elber is a professor in the Computer Science Department, Technion, Israel. His research interests span computer aided geometric designs and computer graphics. Prof. Elber received a BSc in computer engineering and an MSc in computer science from the Technion, Israel in 1986 and 1987, respectively, and a PhD in computer science from the University of Utah, USA, in 1992. He is a member of SIAM and the ACM.

Prof. Elber has served on the editorial board of the Computer Aided Design, Computer Graphics Forum, The Visual Computer, Graphical Models, and the International Journal of Computational Geometry \& Applications and has served in many conference program committees including Solid Modeling, Shape Modeling, Geometric Modeling and Processing, Pacific Graphics, Computer Graphics International, and Siggraph. Prof. Elber was one of the paper chairs of Solid Modeling 2003 and Solid Modeling 2004, one of the conference chairs of Solid and Physical Modeling 2010, the chair of GDM 2014, the conference co-chair of SIAM GD/SPM 2015, and the conference co-chair of SPM 2018. He has published over 200 papers in international conferences and journals and is one of the authors of a book titled "Geometric Modeling with Splines - An Introduction". Prof. Elber received the John Gregory Memorial Award, 2011, in "Appreciation for Outstanding Contributions in Geometric Modeling", the Solid Modeling Association pioneers award in 2016, and the Bezier award in 2019.

Gershon Elber can be reached at the Technion, Israel Institute of Technology, Department of Computer Science, Haifa 32000, ISRAEL. Email: gershon@cs.technion.ac.il, Fax: 972-4-829-5538.

Ilias S. Kotsireas serves as a Professor of Computer Science and Director of the CARGO Lab (http://www.cargo.wlu.ca) at Wilfrid Laurier University in Waterloo, Ontario, Canada. He has over 150 refereed journal and conference publications, chapters in books, edited books and special issues of journals, in the research areas of Computational Algebra, Metaheuristics, High-Performance Computing, Dynamical Systems and Combinatorial Design Theory. He serves on the Editorial Board of 8 international journals. He serves as the Managing Editor of two Springer journals and as the Editor in Chief of a Springer journal and a Birkhauser book series. He has organized a very large number of international conferences in Europe, North America and Asia, often serving as a Program Committee Chair or General Chair. He served as Chair of the ACM Special Interest Group on Symbolic Computation (SIGSAM) on a 4-year term 2013-2017. He is a co-chair of the Applications of Computer Algebra Working Group.

Zoltán Kovács is an assistant professor at The Private University College of Education of the Diocese of Linz, Institute of Initial Teacher Training (Austria), since 2015. Team member of Center of Mathematics Education of Linz at University of Linz, Department of Mathematics Education. Core developer at GeoGebra, since 2010. Author of over fifty published scientific papers and hundred scientific communications (including various software packages) in different international journals and conferences. Topics: Mathematics Education, Automated Reasoning in Dynamic Geometry, Computer Algebra.

Michal Tabach is the head of the Department of Education in Mathematics, Science and Technology at Tel-Aviv University.

“Integrating technology into mathematics education is a challenge I have been facing for three decades – first as a mathematics teacher and designer of curriculum resources, and currently as a researcher. As a young secondary school teacher, I regularly taught algebra and geometry with open digital tools. In parallel, I worked towards developing tasks and resources for teaching and learning in digital environments. Naturally, as I became more involved in research, I focused on learning mathematics in an open digital tools environment. However, it soon became clear that teachers were key to successful digital integration. This led me to my current focus, teacher education. I am now leading projects aimed at helping practicing teachers integrate technology into their practice, taking into consideration their pedagogical-mathematical needs. I have been a lecturer and researcher at Tel-Aviv University for 12 years. Currently, I am head of the Department of Education in Mathematics, Science and Technology at Tel Aviv University.”

Attila Szabo, PhD, is an associate professor at the Education administration in Stockholm and at Stockholm university. He is a board member of the Swedish Institute of Educational Research.

Attila’s research focuses mathematically gifted students and computer programming in mathematics education. He supervises prospective teachers in their degree projects and have taught mathematics in secondary school and at university level. Also, he is author of textbook series for secondary school mathematics.